-
About
Our Story
back- Our Mission
- Our Leadershio
- Accessibility
- Careers
- Diversity, Equity, Inclusion
- Learning Science
- Sustainability
Our Solutions
back
-
Community
Community
back- Newsroom
- Discussions
- Webinars on Demand
- Digital Community
- The Institute at Macmillan Learning
- English Community
- Psychology Community
- History Community
- Communication Community
- College Success Community
- Economics Community
- Institutional Solutions Community
- Nutrition Community
- Lab Solutions Community
- STEM Community
- Newsroom
- Macmillan Community
- :
- Discussions
- :
- The Discussion Board
- :
- Best Practices for Teaching Tricky Topics - Stats
Best Practices for Teaching Tricky Topics - Stats
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Report Inappropriate Content
What topic(s) consistently proves to be tricky for students and how have you overcome this?
- Topics:
-
Innovation in Education
-
STEM
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Report Inappropriate Content
Sampling variation and distributions
An important topic that students have trouble understanding and that is difficult to teach is that statistics computed from random samples vary from sample to sample but there is a well-defined pattern to this variation. Effective teaching paves the way for understanding statistical concepts such as statistical confidence and P-values. In my opinion, recent approaches to teaching introductory statistics using resampling are attractive because students are continuously exposed to these ideas, which reinforces learning.
Visual demonstrations and activities are an effective way to help students learn. When I first started teaching in the late 1970s, we used a bead box to demonstrate this. This was a box full of colored beads with a known proportion of each color. The beads in the box were the population. The statistic of interest was the proportion of beads of a certain color in a sample. The box was shaken and stirred, a wooden paddle with 25, 50, or 100 slots was dipped into the box to take the sample, and the proportion of beads of a particular color in the sample was noted. This was repeated many times and the outcomes recorded.
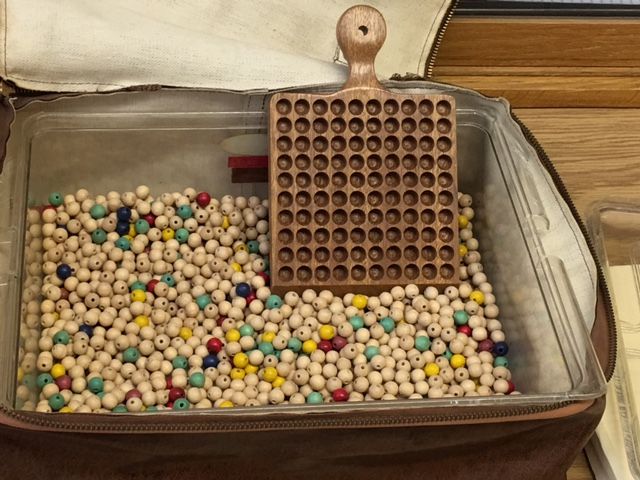
One thing I liked about this was the visual, tactile nature of the demonstration. Thoroughly shaking and stirring of the beads in the box helped convince students that samples were representative and would vary each time a sample was taken. Seeing the paddle with the sample further demonstrated the sample variation. Recording the results in a dotplot or histogram helped students see a pattern emerge (the sampling distribution). If we had several such bead boxes, letting students work in teams to discover this on their own was quite effective.
As time passed, we used tables of random digits, random number generators, or applets instead of the old-fashioned bead box. These can be quite effective, but there are some subtle differences. First, students must take it on faith that generating random numbers with software is equivalent to the physical shaking and mixing of the beads in the bead box. Second, students must recognize that the numerical labels are equivalent to actual objects (beads). If students don’t grasp these abstractions, the whole demonstration becomes something of a black box. I have no idea whether this is a real problem or just an old professor being a bit of a curmudgeon.
Some sort of activity, whether it be a bead box, random number generators, applets, or something else is valuable for teaching about sampling variation and sampling distributions. Having students engage is such an activity, plotting the results, and discovering on their own that a long-term pattern emerges strikes me as a good way to introduce these concepts. One can use repeated sampling later to show how confidence intervals vary from sample to sample (and hence what a confidence level represents) or how test statistics vary from sample to sample (and hence what a P-value represents). The goal is to help students understand that statistical procedures such as confidence intervals and P-values are rooted in sampling variation.

